Vector analysis of linear and angular deceleration in the M-M expt.
I checked in the local library the other day, to pick up a new physics text
book. As expected, it described the Michelson-Morley calculation of different
times for two perpendicularly reflected split light beams to return to
source.
This text happened to be published in 2008, several years after I first showed,
on my web-pages, that the calculation uses the wrong average.
The so-called null result of the Michelson-Morley experiment is that the beams take the same time to return the same distance, irrespective of their different relations to earth velocity thru space.
Of course, the future of physics was based on the experimental result, and not on the misleading calculation, so, in that respect, it didn't really matter. (My own first web-page on the M-M experiment, when I was trying to teach myself special relativity, faithfully followed the calculation error. As well as making a legion of my own mistakes.)
Nevertheless, as I've claimed many times, the calculation used the wrong average, the arithmetic mean, of each two-way journey.
The geometric mean gives the correct prediction that the two light beams
will take the same time to return to source.
This measurement was made possible by the legendary Michelson-Morley
interferometer to synchronise light waves.
My web page, The Minkowski Interval predicts the Michelson-Morley experiment) was devoted to supporting the geometric mean calculation. This another such page, in terms of a vector analysis of the Michelson-Morley experiment.
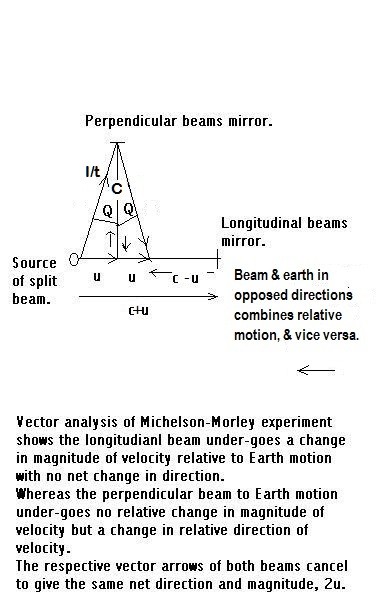
The vectors, on the diagram, are the arrows, which give direction, also having lengths, which give their magnitudes, labeled in terms of the speed of light, c, and earth speed, u.

On the diagram, the light beam is split at source, so that part of the beam is deflected at right angles. Both beams go the same distance before they are reflected back to source.
Meanwhile, the experiment is arranged so that, say, the deflected beam goes at right angles to earth motion, while the original beam continues in line with earth motion. The original beam going against earth motion, before reflection, increases (to c+u) the velocity with which the beam reaches its reflecting mirror. On reflection, going with earth motion decreases (to c-u) the velocity with which the beam goes the same distance back to its source.
Subtracting the longitudinal beam return vector from its out-going vector, or (c+u) - (c-u), gives a net vector of two units in the direction of earth motion, or 2u.
By the time that the deflected beam has reached its reflector, the earth has moved on slightly (with velocity u), which position marked on the diagram is now perpendicular with the deflected beams reflector.
The same thing happens again by the time the deflected beam reflects back to its source which the earth motion has moved on by the same amount (velocity u). So, the deflected light beam has merely moved by two units of earth velocity (2u). These two units are in fact two resultant vectors of the outgoing and return journeys.
The component vectors of the resultant vector, are for the outgoing journey,
the slanting arrowed line of velocity, I/t, pointing towards the reflector, and
the perpendicular line, of value c, the speed of light, pointing towards the
reflector.
The value, (I/t)², is, by theorem Pythagoras, the Minkowski Interval divided by
time, which equals: (c² + [iu]²) = (c² - u²). The operator, i, which is the
square root of minus one, signifies a turn thru 90 degrees. Just as the minus
sign can be an operator for a turn thru 180 degrees, or going in the opposite
direction.
A complex number (composed of an ordinary or real number combined with a
so-called imaginary number, i) like a vector, is a way of expressing direction
as well as magnitude.
Don't be put off by the fact that the longer length hypotenuse, I/t, in the diagram, has a lesser magnitude than the shorter vertical value, c. This is a common-place peculiarity of the representation of the Minkowski Interval radius vector on a plane defined by a complex variable, t(c + iu), respectively on the horizontal and vertical axes.
The perpendicular vector, c, when pointing in the opposite direction, is one
of the two component vectors to the resultant vector for the return journey.
The other component vector is the slanting arrowed line, marked, pointing to
the destination, being the source moved on two units of earth velocity.
This agrees with the resultant vector of the longitudinal beam.
This vector analysis of the Michelson-Morley experiment shows that the split light beams have the same resultant velocity from different component velocities.
Two different kinds of change in velocity affect the split beams. A vector consists of magnitude and direction. The original beam velocity was changed in magnitude by being reflected in line with earth motion. Change in velocity is also called acceleration (or rather decelleration, if a slowing down of velocity, as in this case). The original beam undergoes magnitude deceleration. But the net direction of the original beam remains the same.
The deflected beam velocity was changed in direction relative to earth motion, as shown on the diagram. By convention, angular rotation is anti-clockwise. First, the split beam is rotated by 90 degrees or π/2 radians. The angle of drift with earth motion on reaching the reflector, is minus Q (or positive Q clockwise).
The return beam journey, off the reflector by another angle Q for an equal
amount of earth motion drift, measures an anti-clockwise turn of π + 2Q.
Add this to minus Q, for an overall change in direction of the beam, of angle
π + Q (following its initial perpendicular deflection. This leaves the
deflected beam a remaining angle, of π/2 - Q, from re-alignment with
earth motion).
This directional velocity change is, in other words, directional
deceleration.
There is no reason why magnitude acceleration should be exactly compensated by directional acceleration. It be the result of the particular set-up of the Michelson-Morley experiment.
Directional acceleration and magnitude acceleration are found as the vector components of a resultant acceleration involved in circular motion. In that case, the directional acceleration vectors from the circumference to the center of the circle, and is called normal acceleration. Tangential to that point, there is tangential acceleration, which is the magnitude component of circular acceleration.
Other terms for normal and tangential acceleration, respectively, are centripetal and centrifugal acceleration.
The Michelson-Morley experiment was meant to tell time differences in differently oriented light beams over the same distance. But this vector analysis suggests that the set-up does exactly the opposite. It synchronises the split light beams. That is, it creates a time symmetry.
There must be constants at work that constrain this result. The split beams have the same distance to travel. And both beams are subject to the same constant velocity of the earth.
The experiment can be held for beams at other than perpendicular directions. A beam that is not fully perpendicular to earth motion must, to some extent, partake of the slowing and increasing speed effects of the earth-aligned beam having to chase, or being met by, the reflector.
The Minkowski Interval appears to neatly express the simultaneity of the Michelson-Morley experiment, because the velocity terms can be interpreted as light moving with and against earth motion, or, light moving crossways to it. And that identity, of changes in velocity, constrains the times to be the same.
From the fore-going discussion, it also means that the perpendicular velocity changes involve an equality of angle deceleration to magnitude deceleration.
As tentatively discussed at the end of my page on amplitude symmetry, velocity is usually put in terms of mass, to make momentum. Physicists speak of conservation of linear momentum and conservation of angular momentum.
The Michelson-Morley experiment seems to be a set-up involving the joint conservation of linear and angular momentum. Or, linear-angular momentum conservation.
Richard Lung.
26 june 2011.
Corrections and attempted clarification: 3 dec. 2012; 21 June; 14 nov. & 9
dec. 2013; 28 june 2017.