Differentiation of vector acceleration from the Michelson-Morley experiment and a kinematic-dynamic Interval Equivalence principle.

Michelson-Morley experiment and circular motion.

This page has the modest intention of using conventional differential calculus (as a contrast to my innovation of geometric mean differentiation) in relation to the Minkowski Interval, in the context of the Michelson-Morley experimental set-up.

The following argument relates the Michelson-Morley experimental set-up to considerations of circular motion. Tho intended to test for a universal ether medium of light waves, this experiment essentially tests whether earth velocity makes any difference to the speed of light. This is done by reflecting a light beam in line with earth motion, at the same time splitting the beam at source, so that part of it is reflected at right angles, for the same distance.

The experimenters calculated that the times taken by the two light beams would be different. The physics community was thrown into confusion by the fact that experiment showed the light beams took the same time. But, as I've said on several pages previously, that was because of a simple calculating mistake, or over-sight, in using the arithmetic mean to average the times taken by the reflected beams.

The geometric mean correctly gives the same time taken by the light beams. That is because reflecting the beam with respect to earth motion causes a relative change in velocity, or, in other words, relative acceleration of the light beam parallel to earth motion. (A theory of relativity waited upon Einstein in 1905.) And the geometric mean is the average to use for non-uniform velocity, while the arithmetic mean only serves for averaging constant velocity.

The beam perpendicular to earth motion is also reflected but the reflection makes no difference to the speed of this beam with respect to earth motion. Motion, considered as a vector, includes not only its magnitude or greatness but also its direction.

Altho there is no change in the relative speed of the cross-ways beam, with respect to earth motion, there is a change in relative direction. And this implies acceleration, for which the geometric mean remains an appropriate average to take.
It so happens that the Minkowski Interval has a geometric mean form, which can express the Michelson-Morley calculation, if corrected in terms of geometric means.

Like the Michelson-Morley experiment, circular motion is considered in terms of two perpendicular components of acceleration. These are tangential (or centrifugal) acceleration and normal (or centripetal) acceleration.

Tangential acceleration is angular acceleration multiplied by the radius. Even when there is no angular acceleration, so that the circular motion is uniform, there is still normal acceleration from the change in direction of its velocity.

The Michelson-Morley experiment has some bearing on circular motion.

The light beam (speed, c), reflected in line with earth motion (speed, u), gains speed from a tail-wind boost and loses speed from a head-wind. That is, respectively: (c+u) and (c-u).

Meanwhile, by the time that the transverse beam has reached its reflecting mirror, the earth has moved on (by velocity, u). And by the time it simultaneously meets its partner beam again at the source, the earth has moved on again by an equal amount, u.

What this means is that the cross-ways beam reflecting mirror is not quite perpendicular to the beam source, considering that it has moved on with the earth, a tiny amount, during the transit of the perpendicular beam from source to reflecting mirror.

Likewise, there is an equal angle (say, Q) made by the reflected cross-ways beam back to the source, also moving with the earth.

This is pictured in the diagram on the previous web page: The Michelson-Morley null result as a linear-angular momentum change conservation. It is shown again here for convenience of reference.

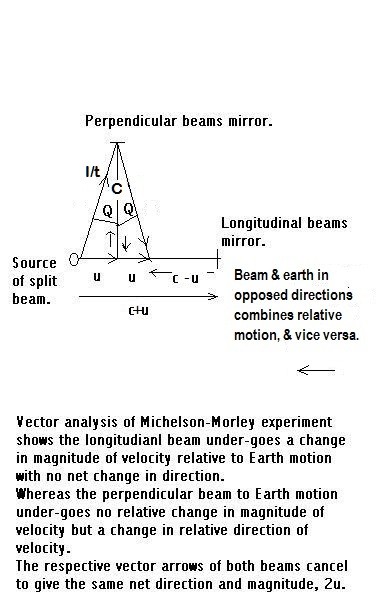
Vector analysis of Michelson-Morley experiment.

The cross-ways beam reflecting mirror may be considered the origin of a circle, to which the cross-ways beam is like a rotating radius.

With reference to the diagram, the split light beam travels from the source, perpendicularly. However, the motion of the Earth (speed u) carries the cross-ways light beam somewhat before it reaches its reflecting mirror. A similar effect is repeated as the crossways light beam is reflected back to the source.

By the way, the diagram very much exaggerates this crossways drift of the light beam, because the motion is actually very slight compared to light-speed and the angle of drift, angle Q, would not be noticeable. It is the genius of the Michelson-Morley interferometer that is able to detect such small effects.

This cross-ways light beam drift can be seen as a summation of three vectors. By the time this light beam, drifting from perpendicular, has reached its reflecting mirror, the Earth has moved on to a position where the source is still perpendicular to the cross-ways reflecting mirror.

A vector is a magnitude and direction.
The first vector is the cross-ways light beam, of magnitude light-speed c, and an arrow pointing from the source to its reflecting mirror.
The second vector is an arrow pointing from the reflecting mirror perpendicularly to the source, that has moved, at the rate of Earth velocity u, which is the third vector and summation vector of the first two vectors.
The third vector, of magnitude u, has the direction from the original source position to the position of the source when the cross-ways beam reaches its reflecting mirror.
Similar considerations apply for the journey of the reflected cross-ways beam.

The second vector can be calculated by the Pythagoras theorem, where the light beam velocity, c, is the hypotenuse:

The square of the second vector equals: c² - u². This happens to be the square of the Minkowski Interval, I, divided by time, t, squared. Or:

(I/t) = (c² - u²)^1/2.

This formula comes into effect for velocities significant relative to light speed. In this case, it is the velocity of a light beam cross-ways relative to earth motion.
The same working applies for the reflected beam which is also moving cross-ways, at the same rate, relative to earth motion.

Obviously, whatever average (such as arithmetic mean or geometric mean) one took of the cross-ways relative velocity before and after reflection, because they are the same, the average would also be the same.

Again with reference to the diagram, the light source is shown in three positions: its original position, when it emits the perpendicularly split light beam; its second position perpendicular to the cross-ways light beam about to be reflected; and its third position when both beams have been reflected to source, which the Michelson-Morley interferometer showed to be at the same time.

The two source transitions are lines both given by Earth velocity u. In sum, they are a line of magnitude 2u. This line makes a chord to a circle segment, of radius light beam speed c, swept by the two angles, Q, or 2Q.

Merely differentiating the earth velocity, u, would be a classical or non-relativistic differentiation. A relativistic differentiation requires calculating in terms of a relativistic velocity. To calculate in terms of relativistic velocity, the Earth velocity must be considered relative to the light beam velocity. With respect to the earth-longitudinal light beam, this involves the average velocity of the light beam reflected both ways in relation to the direction of Earth motion.

In other words, relativistic velocity is another name for geometric mean velocity. The theory of relativity is a theory of geometric means for averages.

The light beam reflection changes its velocity relative to Earth motion, Changing velocity (rather than constant velocity) implies it is unsuitable to use the arithmetic mean used in the original calculation by Michelson and Morley.

Instead, the geometric mean must be used. For the Earth-longitudinal light beam, this gives the square root of the velocities with and against Earth motion, respectively, (c+u) and (c-u). Hence geometric mean longitudinal velocity is:

{(c+u)(c-u)}^1/2 = I/t. This is the Interval divided by time.

The same geometric mean velocity was obtained, above, using Pythagoras theorem, for the cross-ways journey of the perpendicular light beam to the longitudinal light beam.

Since the split light beams have the same average velocity (that is using the geometric mean instead of the arithmetic mean) then they must take the same time to return to source. And that correctly predicts the result of the Michelson-Morley experiment.

Thus the relativistic equation of the Minkowski Interval is the geometric mean distance. When divided by time, it is geometric mean velocity.

Relativity seems to mean averaging. In classical physics, the relativity principle seems to involve the arithmetic mean. But in high-speed or high energy physics, relativity seems to mean averaging by the geometric mean.

Conventional differentiation of perpendicular relative velocities.

To top

The average velocity, the geometric mean, for the longitudinal journey is:
{(c+u)(c-u)}^1/2 = (c² - u²)^1/2 = cg = I/t.

The symbol, g = (1 - u²/c²)^1/2, has the form of the gamma factor, originally called the Fitzgerald-Lorentz contraction factor. In the context of the Michelson-Morley experiment, where the two observational times are the same, it actually is the gamma factor. But in the Minkoski Interval, this isnt generally the case: What I call "the Minkowski factor", g, is more general than the gamma factor.

And (from my point of view) the gamma factor was essentially a correction for the fact that the physics tradition, following Michelson and Morley, have used the arithmetic mean, when (according to me) the geometric mean is required to average the reflected journeys of the perpendicular light beams.

This contention of mine may be relevant to other interferometer experiments that have descended from Michelson-Morley, whose names in technical achievement are as legendary as Archimedes. For instance, there are modern laser interferometers that attempt to detect gravity waves. In so far as they follow the classical technique of averaging the time taken by reflected lasers, in relation to, say, a gravity wave (instead of in relation to the earth) then I guess this raises the same objection to use of the arithmetic mean, rather than the geometric mean, to average relative acceleration of a reflected laser, since the reflection would change the velocity of, or accelerate, the laser light, in relation to the motion of earth or a gravity wave or whatever.

I know little physics and less maths but I'll attempt a run-thru of a conventional treatment of the differential calculus of geometric mean velocity.

The Interval is a constant, it is the common "space-time" measure that all observers of a given event, arrive at from their differing local measures of the events space and time co-ordinates.

The Michelson-Morley experiment deals in constant velocities, wheher of light or the earth motion, and measures a constant time, shared by the return journeys of the split light beams. Therefore, there would be no acceleration to consider in these terms.

Departing from the classical Michelson-Morley experiment, the light beams might have a carrier, other than Earth, whose velocity changes over time. Given that variable, v is dependent on independent variable, t, it should be possible to differentiate.

The differentiation from first principles is given here (as distinct from my new differentiation of a geometric mean derivative, given elsewhere).
Instead of the Greek letter delta, I use the hash sign to stand for a (usually small) change in the variable:

Let #t approach a limit of zero. And express the resulting differentiation by replacing the hash sign of a change-variable, #, with symbol, d:

This derivative has the dimensions of (a change in a change of) distance per time squared; or a change in velocity over time, or, acceleration, a.

Coming to the cross-ways velocity, it is of the same magnitude, v, as the longitudinal velocity but it is not simply taking place along a line, but at two angles, Q, over a plane. This circular motion might be calculated from trigonometry. Let Q = wt, where w means angular velocity. Thus (from the diagram):

By standard rule of trigonometric differentiation, its derivative is: dv/dt = -cw sin wt.

However, some care is needed here to determine whether the two derivatives, of v with respect to t, are actually the same:

If velocity equals angular velocity times radius, v = wr, then apparently radius, r = ct. From the diagram, marked in velocity terms, the radius appears as light speed, c. Radius, as a distance dimension, is estimated by multiplying velocity (in this case, c, the lengths of both lines to the angle 2Q, in the diagram) by time, t. Hence, radius, r = ct.

In that case, therefore, the two versions of dv/dt are only equal when: sin wt = 1. The sine of an angle is only equal to one, at angle Q = π/2 or ninety degrees, and every full turn of 2π or 360 degrees thereafter. This would be when, in the diagram, u = c. This is consistent with sin Q = u/c.

Moreover, if u = c, then the third side of the triangle, I/t = {(c²-u²)^1/2}t/t = 0.

This seems to be an occasion in which it is useful to think of Special Relativity in terms of averages. The reflected cross-ways light beam is still, on a plane, in two dimensions, and the reflected longitudinal light beam is still on a line, in one dimension. But for each of them, on average, their geometric mean, that is the Interval, is zero.

Vector Averages: the geometric mean vector.

The perpendicular beams should have a resultant vector acceleration, of a direction in between the two, and of magnitude given by Pythagoras theorem.

The vector acceleration is light velocity over time, c/t, times two geometric means with ranges at right angles to each other, as signified by velocity, u, having the imaginary coefficient, i, in the second factor.

In terms of my unorthodox way of thinking, the Minkowski factor, g = (1 - u²/c²)^1/2, is not so much a correction factor or "shrinking factor" but a geometric mean or average over a range from limits of (1 - u/c) to (1 + u/c).
Similarly, its imaginary version, is a geometric mean over a perpendicular range with limits from (1 - iu/c) to (1 + iu/c), where i, the square root of minus one, serves as an operator for moving a value thru ninety degrees (just as a sign of minus one, which equals i², familiarly moves a value thru a further ninety degrees, that is in reverse, or negative, direction of one hundred and eighty degrees).

This represents vector acceleration in terms of complex numbers. In mathematics, complex numbers and vectors are recognised as two ways of representing the same thing.

Here then are now two ranges. Not only from (c-u) to (c+u) but another range from (c-iu) to (c+iu). The only difference between u and iu is that the imaginary version of u is at right angles to the so-called real version of u. The imaginary coefficient signifies the operation of turning value, u, thru ninety degrees.

If light speed, c, was the value at the pivot of a compass, then the velocity u, by its four signs is at the four points of a compass, north, iu; east, +u; south, -iu; and west, -u.
The light speed is at the center of these four directions of variation in speed, u. And of course these variations of velocity, about the light speed, are on a plane.

(All the possible positions on a plane are mapped by complex numbers, which are a co-ordination of a real number plus an imaginary number. The mappings of the four points of the compass are also combined numbers but their partner ordinate numbers happen to be zero, so they are left out. The origin of a graph, with which the four compass points are in line, is usually set at zero. Typically the value of the origin is adjusted to zero for convenience, just as light speed itself is adjusted, usually to one or unity for convenience.
These conventions are meant to simplify, tho they take a little explaining.)

Previously, the geometric mean was explained as an average or representative value of a range of values along a line or single dimension. But in this case, the geometric mean can be conceived as a (vector) average of two lines of ranges, at right angles to each other, and hence representative of two dimensions or a range about a plane.
Hence, the geometric mean vector

In other words, this compass-like vector of velocities varying, at a given level, in all directions about light speed, is a two-dimensional complex velocity. Not to forget that it is divided by time, t, which makes for a complex acceleration, A. That is to say, the equation for vector acceleration, A may be considered as a complex geometric mean acceleration.

Moreover, motion in a circle, say, of a spinning compass, translates into wave motion, where the distance from north to south is the amplitude of a wave from crest to trof. But when the variable is complex for mapping a plane, rather than a line, the wave wouldnt be like the up and down motion of a piece of string attached to a wall at one end and given a fillip by someone holding the other end. It wouldnt be a simple vibration up and down in one vertical dimension. Rather it would be a planar vibration.
(A cork-screw is a possible evolution of a planar vibration.)

However many dimensions these wave motions are measured in, they give a picture of the Interval as being of observers whose local measures differ from one another in terms of their differing local velocities, as differing amplitudes varying about an equilibrium of light speed.
Tho, taking their (geometric mean) average ensures no (massive) object quite reaches light speed.
From the mathematical form of the Interval, locally measured observer velocities look like tachyonic crests and tardyonic trofs statisticly ranging about that equilibrium we know as the constant speed of light.

The concept of mass is the province of dynamics and this discussion has been in terms only of kinematics, whose equivalent term is time. In terms of time, rather than mass, no acceleration ever slows down time sufficiently to reach timeless light speed.

It has been said that special relativity is a new theory of absolutes, like classical physics but replacing an absolute speed and time and velocity (of the ether) with an absolute speed of light. But the above analysis suggests that this is not unconditionally the case. The geometric mean of speeds relative to light speed is something of an equilibrium speed whose amplitude varies as the speeds of local observers.

This interpretation of large-scale physics is supported by quantum electro-dynamics (QED) of the extremely small distances in which light varies in speed above and below the normal speed it averages out at.

The Michelson-Morley experiment with its split perpendicular light beam reflections might be thought of as a single perpendicular oscillation. If these reflections were repeated, then the experiment would be reminiscent of the fact that light itself is a perpendicular electro-magnetic wave oscillation.

A typical wave equation is of two second order derivatives. Relatively speaking, this is what are the two accelerations for longitudinal reflection and cross-ways or transverse reflection, given above. Usually the waviness (down the crests and up the trofs) is dependent on time and distance variables. (As well as distance having a wavelength, time has a wavelength called the period.)

In the above example, the independent variable for distance is the angle Q. Hence differentiating, from v = c cos Q. Then dv/dQ = -c sin Q = -cu/c = -u. Assuming some unknown coefficient (given by question mark, ?) is needed to equate dv/dt to dv/dQ.

And (without using the curly letter-d customary for signifying partial differentiation, involving more than one independent variable):

Or expressed as a second order equation of the Interval distance over a change in a change of time, t, and change in a change of the angle Q:

In the equation of (geometric mean) average velocity, v, which equals c(1-u²/c²)^1/2, this velocity changes, meaning there is acceleration. Therefore, velocity, u, must change (light speed, c, being constant). In the Michelson-Morley experiment, velocity u, is the earth velocity, which is practically constant. So, another situation would have to be imagined of an earth or carrier that changes velocity.

In the Minkowski Interval, velocity u, is the local velocity that any given observer measures in their frame of reference. All observers agree on the Interval, the combined space and time measure of a given event, tho their space, time and velocity measures differ from their different view-points.

Conventionally, in Special Relativity, the Minkowski Interval only applies to constant velocity or motion in a straight line. There wouldnt be much point in differentiating observers local velocities, u, because this is liable to lead to non-relativistic derivatives. Instead, the relativistic velocity, v, must be differentiated and this should produce the sort of relativistic acceleration differentiation shown above.

The Interval itself remains constant, thru that differentiation, as the co-ordinating measure that allows different observational reference frames to agree on their measure of an event.
(This is possible from differentiating v = I/t, where I is constant, to obtain an acceleration in terms of: dv/dt.)

But a further change is allowed. Not only may observers measure different local velocities but the local velocity of each observer may now itself change (which goes beyond the popularisations of special relativity, with which I am familiar).

I admit unanswered questions, such as that acceleration implies an Einstein equivalence with gravity and curvature of space and its Riemann geometry. While the Minkowski Interval is just an extension of Euclid geometry of flat space.

(A BBC Horizon program of 3 September 2012 confirmed that astronomy measures the universe to be flat space. They triangulated Earth onto the opposite edges of a random heat splodge in the microwave radiation background left over from shortly after the Big Bang. And the triangle added up to 180 degrees.)

The Equivalence Principle and kinematic-dynamic Intervals equivalence.

This last section is just an after-thought, which Ive tagged-on for good (or ill) measure. Mainly in ignorance, it suggests but does not show or demonstrate. My apologies, as ever.

Einstein's Principle of Equivalence of acceleration to gravity is the basis of the General Theory of Relativity. It would seem reasonable to suppose that in so far as acceleration is pre-figured in Special Relativity, so should be the Equivalence Principle.

Conventional differentiation belongs to a mathematical field called linear analysis. For instance, it is not suitable for non-linear analysis such as involved in Chaos theory.
And Einstein's field equations of general relativity are non-linear, which also makes them difficult to solve.

From what I could gather, conventional differential calculus, as linear analysis, at least, needed adapting for the curvilinear geometry that replaced the linear geometry of the Minkowski Interval, essentially a four-dimensional extension, to a so-called space-time, of Euclid's straight-line geometry, in three-dimensional space, and suitable for describing uniform motion in a straight line.

Apart from this geometric generalisation from Euclid and Minkowski geometry, as a geometry of zero curvature, Einstein broke radically with special relativity in deriving general relativity.

The basis of this radical departure is Einsteins famous thought experiment, of the accelerating lift in outer space, produces the effect of light bending at fantastic accelerations, as it crosses from one window to another, that would appear to an observer inside the lift, as he also undergoes a gravitational-type fall. Hence the equivalence principle of acceleration to gravity. And the need for a geometry of curvature to describe the bending and the replacement of a supposed force of gravity with the notion of space and time curving.

Having admitted those limitations to conventional differentiation generalising special to general relativity, still, an element of the acceleration frame of reference might be pre-figured in special relativity. And it may be that so is the principle of equivalence. I am just supposing!

Einsteins equivalence principle does not actually say that acceleration equals gravity. Acceleration belongs to kinematics, the study of motion. But gravity or gravitational mass belongs to the further field called dynamics.

The version of the Interval, given above, is the kinematic version. But it also has a dynamic version. This is completely analgous to the kinematic version, except that time is replaced by mass. Instead of time multiplied by velocity to give a distance variable, velocity is multiplied by mass to give momentum, p. And instead of light speed multiplied by time, light speed is multiplied by mass, and usually expressed in terms of energy, E, using the famous equation of Poincare and Einstein, of E = mc².

Thus there is a sense in which there is an equivalence between the kinematic and dynamic Intervals. If the time and mass variables happened to be the same, they would in fact be equal. At any rate, time and mass are in proportion in equating the kinematic and dynamic Intervals.

So, besides differentiating a velocity by time for an acceleration, it would be equally possible to differentiate that velocity in terms of mass, which varies proportionately to time. Considering mass as gravitational mass, then here may be an equivalence between gravity and acceleration, the basis of general relativity, derived from special relativity.

Richard Lung.
2011 & (briefly uploaded before revision) 28 july 2012;
30 december 2012.

Conventional Differentiation from the Michelson-Morley experiment: Vector Averages.

Michelson-Morley experiment and circular motion.

Conventional differentiation of perpendicular relative velocities.

Vector Averages: the geometric mean vector.

The Equivalence Principle and kinematic-dynamic Intervals equivalence.