The Michelson-Morley experiment was perhaps as revolutionary a chronometer as the salvaged mechanical planetarium attributed to Archimedes. Michelson-Morley interferometer used a synchronisation of light waves to tell the time. These are engineers of genius in their ingenuity. These are the people who make a difference.
The Michelson-Morley experiment found that perpendicularly split light beams
relative to Earth motion took exactly the same time to be reflected back to
source over the same distance.
They calculated that the longitudinal beam, with respect to Earth motion, would
take longer than the transverse beam. As far as I know, no one in physics has
ever questioned the correctness of this calculation. At least that is what I
was told by a physics moderator, who refused to post my alternative suggestion.
Before the kind reader automatically assumes, like the good moderator, that
I must be wrong, recall the letters of Richard Feynman, Don't You Have Time To
Think? edited by his daughter.
A woman wrote to ask why she was told she was wrong, when she was just
following the Feynman lectures. Feynman replied: Because he was wrong on this
point. We goofed. That will teach you not to follow authority.
The point about the Michelson-Morley calculation is that it is based on averaging the times taken by each beam over both the back and forth part of its journey. The average used for the longitudinal return journey was the arithmetic mean. As I've said, more times than I can remember, the geometric mean is the average that gives the correct prediction.
In special relativity, massive objects significantly approaching
light-speed, need disproportionately more energy, the closer they get to that
limit. The path of this deceleration could be mapped out as a geometric series,
averaged by the geometric mean. So there is nothing odd about using the
geometric mean in the context of special relativity.
Of course special relativity hadnt been invented by the time of the
Michelson-Morley experiment, which indeed was to lead to it.
The Minkowski Interval has a geometric mean form compatible with the
Michelson-Morley experiment result. Whereas the original Michelson-Morley
calculation, applied to the Lorentz transformations and the Interval, I found,
don't compute.
Also, the perpendicular light beams equal times can be demonstrated by vectors,
as I formerly did, using figure 1.
So why am I laboring this point over and over again? I didn't mean to. I just stumbled on a little more supporting evidence: item four, in my case. In any case, I think Ive already made the point sufficiently well. It is up to the physics community to come to terms with it, one way or the other. They have had plenty of time.
It is true, I am not one of them. I have not received a natural science training and wouldnt have stayed the course. I only just managed an undistinguished social science qualification, really a disqualification from doing any academic research – mercifully. And that is why I cannot be sure about this further demonstration that the geometric mean is required for calculating the Michelson-Morley experiment.
But - apparently - the experiment is an example of the Principle of Least
Action, which describes none other than the geometric mean. Physicists are
hardly likely to abandon one of their most important principles, so it does
seem it would be reasonable of them to grant that the geometric mean is the
right, tho not the historically used, average in calculating for the
experiment.
It's only a small and historicly understandable, if time-honored over-sight by
the physics community.
I make bigger mistakes than that, on an on-going basis, some of which I noticed
on my web pages, to go back and correct. I apologise for my errors. I trust my
own authority in physics much less than that of the physicists.

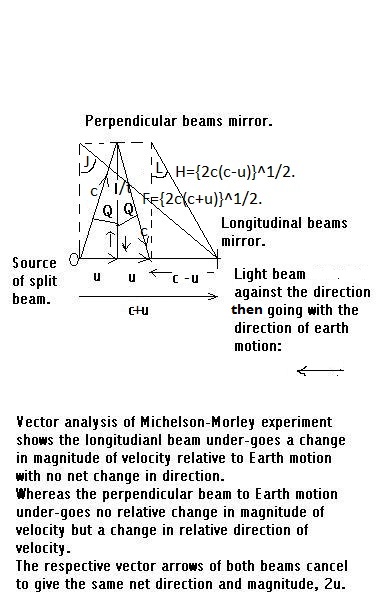
Figure 1 is a schematic representation of the Michelson-Morley experiment. The drawing is not to scale. The earth speed, u, has been greatly exaggerated in comparison with light speed, c, so that it shows at all. The light beam is split at source (on the lower left of the picture). The horizontal part of the beam, at light speed, c, goes against the earth motion, u. This gives the speed, c+u, because earth and beam are coming to meet each other, so their speed is combined. But when reflected (on the lower right of the diagram) the speed with the direction of earth motion, is c-u.
The out-bound speed, c+u, is co-terminous with the angle, J (in the top left corner of diagram). The vertical side, of the triangle formed, is I/t. This also has the dimensions of velocity, being a distance constant, the Minkowski Interval, I, divided by the time, t, which is a local time unique to any observer of a given event, significantly approaching light speed.
The Minkowski Interval was not invented till many years after the Michelson-Morley experiment. It is the agreed measure of an event by all physical observers, generalised from their local length and time measures.
I = t(c² - u²)^1/2 = t'(c² - u'²)^1/2.
The repetition of the Minkowski Interval, with the apostrofes, merely signifies a second local observer with different local time and velocity, when he measures the same event. The equation shows, per observer, only one velocity, which may signify one dimension or a vector of two or three dimensions combined. The point is that both, and indeed all, observers measure the same Interval, I, for an event.
Here, the concern is simply with a velocity, I/t = (c² - u²)^1/2.
Pythagoras theorem can be used to calculate the hypotenuse velocity, F (on the diagram): Its square is the sum of the squares of the other two sides.
F² = (I/t)² + (c+u)² = (c² - u²) + (c+u)²
= (c+u)(c - u + c + u) = 2c(c+u).
Therefore: F = {2c(c+u)}^1/2.
The same procedure is followed with respect to the returning beam velocity of c-u. The extent of its line, on the diagram, is co-terminous with angle, L. The right-angled triangle formed has a hypotenuse, H, also found by Pythagoras theorem.
H² = (I/t)² + (c-u)²
= (c² - u²) + (c²+ u² - 2uc)
= 2c² -2uc.
H = {2c(c-u)}^1/2.
The next step is to take the squared sines of the angles, J and L.
Convention makes (sin J)² = sin²J.
sin²J = (c+u)²/2c(c+u) = (c+u)/2c.
Likewise:
sin²L = (c-u)²/2c(c-u) = (c-u)/2c.
Therefore:
sin²J + sin²L = (c+u)/2c + (c-u)/2c = 1.
Unity is the over-all ratio of earth-relative light velocity to
earth-independent light velocity.
I label this a total energy equation. Total energy adds kinetic energy, or
energy of motion, to potential energy, which may be likened to stored
energy.
On a roller coaster, when the car goes up-hill, it is slowing down, losing kinetic energy, but gaining potential energy. The (c-u)/2c term, in the total energy equation, is equivalent to the up-hill motion, because it is losing energy of motion, in chasing earth motion, taking its objective away from it, but storing-up energy for the return journey, when its relative velocity increases, with the earth carrying its objective towards it, the condition given in the (c+u)/2c term.
Therefore, (c+u)/2c corresponds to a kinetic energy term and (c-u)/2c corresponds to a potential energy term.
Another energy condition (called the Lagrangian) is the kinetic energy minus the potential energy. The Hamilton principle of least action established that, in a field of force, a freely moving body will graph a Lagrangian over time, that minimises the area under the curve.
This area is found by integration (or anti-differentiation) over time, from start to finish.
Thus, subtracting the kinetic and potential energy terms:
sin²J - sin²L = (c+u)/2c - (c-u)/2c = u/c.
This is the ratio of earth velocity to light velocity.
From figure 1, u/c = sin Q.
This angle measures the amount the transverse light beam, in the Michelson-Morley experiment, drifts with earth motion, on its way to the perpendicular reflector.
To integrate, which means anti-differentiate, sin Q, with respect to time, sin Q = sin wt, with angular velocity, w, and time, t. Trigonometric integration, by rule in calculus, derives: -w.cos wt.
Using the dollar sign, $, for the traditional long-s to symbolise integration or s-for-summation, and to show the integration takes place with respect to changing time, dt, then:
$ sin wt. dt = -w.cos wt.
From Figure 1,
cos Q = cos wt = (I/t)/c =
I/ct = {(c²-u²)^1/2}/c.
The geometric mean, of (c+u)/2c and (c-u)/2c, considered as a true sample of
values for some path to be appropriately represented or averaged, is given by
multiplying them and taking their square root.
This geometric mean equals:
{[(c+u)/2c] x [(c-u)/2c]}^1/2
= {(c+u)(c-u)/4c²}^1/2.
= {(c² - u²)^1/2}/2c.
= I/2ct
To make the least action result, for the kinetic and potential terms, equal the geometric mean of the terms, the appropriate angular velocity, w, must be chosen. This turns out to be minus one-half: w = -1/2.
For, the calculus rule for the integral of sin wt is: -w.cos wt =
-(-1/2)I/ct = I/2ct.
Thus the geometric mean of the kinetic and potential energies is equated to the
least action integral of their (Lagrangian) subtracted values.
It is also necessary to check whether the value, w = -1/2, in Q = wt, is not arbitrary but consistent with the rest of the system.
This can be done by finding the other angular velocities of angles J and L. Say, their angular velocities are k and n, so that J = nt and L = kt. The Michelson-Morley experiment says the times for the return journeys of the split light beams are the same.
I briefly repeat my dissenting reason why this is the logical result as well as the experimental result. The perpendicular light beams go over the same distance. Equate this distance, the same both ways, to the Minkowski Interval, which is also a constant and in the appropriate dimension of distance.
The calculation for the transverse part of the split light beam, from source to reflector, uses Pythagoras theorem to give a velocity of (c² - u²)^1/2. Divide this into the distance, I, for the time, t. because I/(c² - u²)^1/2 = t. The effect of earth motion, on the transverse beam, is the same for the journey back from reflector to source. So it doesnt matter what average is used to estimate the time for the whole journey, it will still be the same time, t.
That is why the historical Michelson-Morley calculation for the transverse
journey is bound to be correct.
For the longitudinal light beam relative to earth motion, it does make a
difference which average is used to estimate the time. That is because, on one
direction of the beam journey, it has the earth effectively as a tail-wind to
light speed, namely c+u. Whereas on the other half of the journey, the light
speed is effectively in a head-wind against earth motion, u, and has an
effective speed of c-u.
This means that the reflection of the longitudinal light beam changes the relative velocity of earth and light beam. There is effectively an acceleration or change in velocity. But the arithmetic mean, used in the historic calculation, is only suitable for a velocity, not a change in velocity, which requires the geometric mean and which confirms the experimental result.
The geometric mean velocity of the longitudinal return journey is the square
root of the multiple of the two velocities, or, {(c+u)(c-u)}^1/2.
To get the time, divide the distance, I, by this geometric mean velocity. This
gives I/{(c+u)(c-u)}^1/2 = t. This is the same time taken as the transverse
journey. Theory supports experiment.
Having established a common time for angles, Q, J and L, it should now be straight-forward to find their respective angular velocities. This is done by making use of the fact that the three angles are all in right-angled triangles and they all have an adjacent side of common magnitude, I/t.
So, taking the cosines of each angle:
Cos Q = (I/t)/c = cos wt = cos (-1/2)t.
Cos J = (I/t)/{2c(c+u)}^1/2 = cos kt.
Taking the ratio of Q/J:
[{2c(c+u)}^1/2]/c = k/(-1/2).
Therefore:
k = -[{2c(c+u)}^1/2]/2c
= -{(c+u)/2c}^1/2.
A similar procedure, for angle L = nt, establishes that: n = -{(c-u)/2c}^1/2.
So, the squares of the angular velocities, k and n, of J and L repeat the respective squares of the sines of J and L, which, added or subtracted, formed the two energy conditions, on which was based the reasoning that the Michelson-Morley experiment satisfies the principle of Least Action.
However, the result of I/2ct looks a bit odd. Why the one-half? It was reached as the integral of sin Q. But angle 2Q represents the arc of the full earth-drift of the transverse beam. Hence, twice the integral of sin Q gives 2I/2ct to make I/ct for a transverse light beams full reflected journey.
In the Michelson-Morley experiment, this is the same measure as for the
earth-longitudinal beam. Both beams cover the same distance, I, over the same
time, t.
Since the times are the same, in this special case, I/ct reduces for both
beams, to:
{(c²-u²)^1/2}/c = (1 - u²/c²)^1/2.
This is recognisable as the contraction factor, meant to harmonise the
discrepant times of the Michelson-Morley calculation, not realised by the
experiment.
Its significance perhaps is as a ratio, of the light beams earth-relative
velocity, to lights earth-independent velocity. That suggests a distinction
between local velocity and universal velocity of light.
My contention has been that all the contraction factor does is introduce a geometric mean that should have been in the original calculation. For, the contraction factor has geometric mean form.
And now, I make a more tentative suggestion that instead of thinking in terms of an ad hoc shrinking factor, it might be more principled to think in terms of least action.
The principle of least action is used to predict future positions of objects, either in classical physics or quantum physics. In the latter, the more or less probable positions of sub-atomic particles can be determined.
To my mind, special relativity is tantalisingly like the quantum procedure. It seems that the former also uses the principle of least action. And formally faster than light components of the Interval and Lorentz transformations hint at the quantum condition whereby a quantum particle can exist everywhere, including at distances not limited by the light speed barrier.
(Quantum theory still does not allow any super-luminal transfer of information, so is still constrained by the basic principle of special relativity.)
This multitude of possible positions are each denoted by a vector and their conflicting directions are responsible for most of them canceling each other out, to give an appearance of following the classical laws of motion, except in abnormal situations which give away the underlying game. Richard Feynman explains this in his popular book on QED.
Mostly self-canceling light vectors straighten the path and average the speed of light, to its familiar constant motion.
The classical scale Michelson-Morley experiment also involves largely self-canceling light vectors. The earth-relative faster-than-light speed vector, (c+u), is sufficiently canceled by a directly opposite slower-than light beam vector, of magnitude, (c-u), chasing earth motion, like a head-wind effect.
The arithmetic mean would average out these two vectors as equal and opposite, exactly canceling each other, to an average of light speed. This does not match physical observation, rendering the arithmetic mean an unsuitable average to use here.
The geometric mean of these two opposite-moving magnitudes, largely cancel
out any relatively moving mass, like the earth, but so their average never
quite reaches light speed..
Brian Cox and Jeff Forshaw (2011): The Quantum Universe. Everything That Can
Happen Does Happen.
Richard Lung.
31 december 2012;
correction 9 december 2013. Re-written with corrections, july 2015; minor
revision may 2018.